InAs / In_0.4Ga_0.6Sb superlattice dispersion with 8-band k.p (type-II band alignment)

Authors: Stefan Birner, Michael Povolotskyi

Input Files:

• T2SL_InAs-GaInSb_Grein_JAP_1995_1D_nnp

This tutorial aims to reproduce Fig. 2(a) of “Long wavelength InAs/InGaSb infrared detectors: Optimization of carrier lifetimes” by Grein and Young.

Conduction and valence band edges

The heterostructure is a superlattice with 3.98 nm InAs and 1.5 nm In_0.4Ga_0.6Sb, where both constituents are strained with respect to the GaSb substrate.

The structure has a type-II band alignment, i.e. the electrons are confined in the InAs layer, whereas the holes are confined in the In_0.4Ga_0.6Sb layer.

The In_0.4Ga_0.6Sb layer is strained pseudomorphically with respect to the GaSb substrate, leading to a compressive strain (-2.5%) which splits the degeneracy of the heavy and light hole band edges in this layer. Thus, the heavy hole band edge lies above the light hole band edge.

The InAs layer is also strained pseudomorphically with respect to the GaSb substrate, and is thus under slight biaxial tension (+0.6 %). The splitting of the hole band edges is the opposite as in InGaSb, i.e. the light hole band edge is above the heavy hole band edge.

The following figure shows the electron and hole band edges.

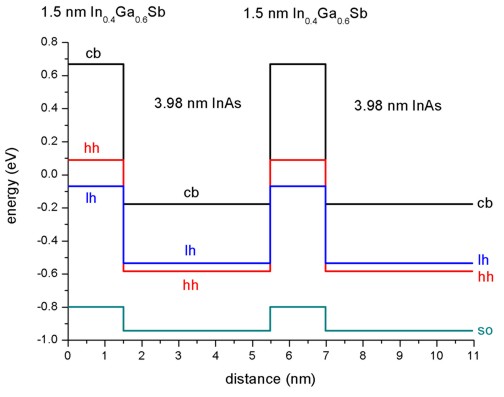
Note that the origin of the energy scale is set to the GaSb valence band edge energy.

Electron and hole wave function for \(k_{||}=0\)

We simulate one period only (i.e. from 0 nm to 5.48 nm) and solve the Schrödinger equation with periodic boundary conditions to mimic an infinite superlattice.

The following figure shows the conduction band edge and the heavy, light and split-off hole valence band edges in this superlattice structure together with the electron (c1), heavy hole (hh1) and light hole (lh1) energies and wave functions (\(\psi^2\)), calculated within 8-band k.p theory.

One can clearly see that the electron state (c1) is confined in the InAs layer (right part of the figure), whereas the heavy (hh1) and light hole (lh1) states are confined in the In_0.4Ga_0.6Sb layer (left part of the figure).

We used the same material parameters as given in the above cited paper by Grein et al., apart from the \(\mathbf{k} \cdot \mathbf{p}\) parameters.

Electron and hole energies for \(k_{||}\neq 0\)

The following figure shows the E(k_||) dispersion of the electron ground state and the two highest hole states along two different directions in (\(k_x\), \(k_y\)) space.

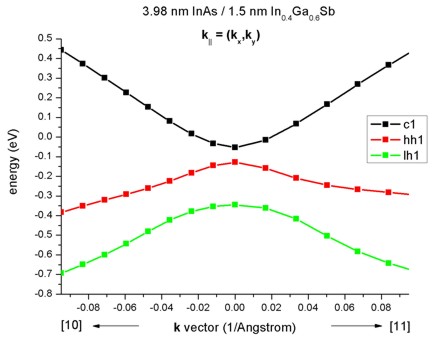
This data is contained in this file: Schroedinger_kp/par1D_disp_01_00_11_hl_8x8kp_ev_min001_ev_max010.dat. Note that the band gap is not determined by the band gap of one individual layer. It is determined by the electron ground state in the InAs layer, and the hole ground state in the InGaSb layer. This means more freedom for band gap engineering.

Electron and hole energies for \(k_z \neq 0\)

The input file used is 1DInAs_InGaSb_k_superlattice_nnp.in

The right part of the following figure shows the E(\(k_z\)) superlattice dispersion of the electron ground state and the two highest hole states. \(k_z\) is the superlattice vector between 0 and 1 \(\frac{\pi}{L}\) where L = 5.48 nm is the length of one superlattice period. (\(1 \frac{\pi}{L} = 0.0573 1/\text{Å}\))

This data is contained in this file: Schroedinger_kp/8x8kp_dispSL_hl_qc001_evmin001_evmax016.dat

The left part of the figure shows the E(\(k_{||}\)) dispersion along [10], i.e. from (\(k_x\), \(k_y\)) = (0,0) to (\(k_x\), \(k_y\)) = (-0.1,0) which is shown in the figure above already.

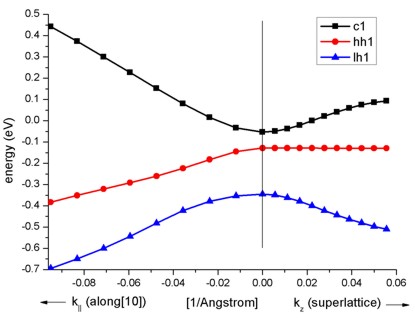
One can clearly see that these heterostructure bands are highly nonparabolic.

nextnano3

Using 1DInAs_InGaSb_SL_k_parallel_superlattice.in, one can generate a 3D plot of the energy dispersion E(\(k_x\), \(k_y\), \(k_{\text{SL}}\)) for each eigenvalue. The files are called dispersion_k_parallel_k_SL_ev001.fld where ev001 indicates eigenvalue number 1.

Last update: nn/nn/nnnn